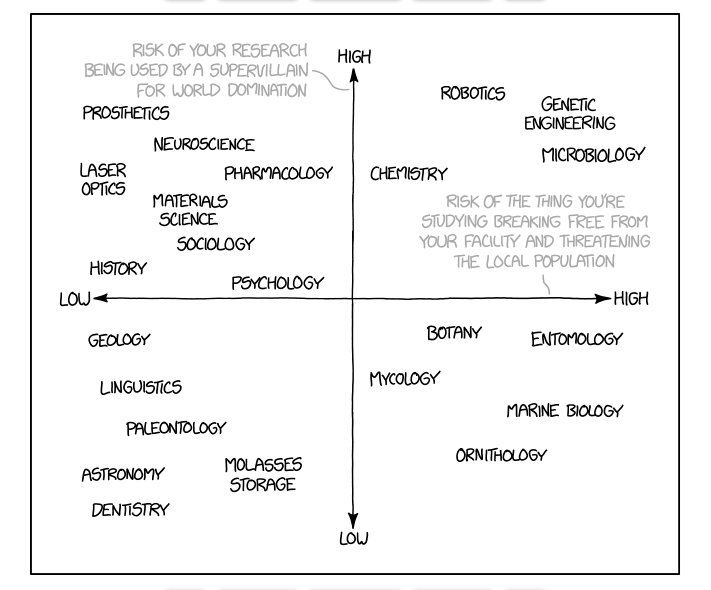
In the last couple of weeks, I’ve been busy preparing for our summer paper on Science Communication. Looking for something amusing about ‘risk’ in science, I came across this neat xkcd.com cartoon about why so many people come knocking on my door (or phoning me, or emailing me) desperately wanting me to spend hundreds of thousands of dollars proving their sensational, Nobel-prize winning, world revolutionizing, if-only-someone-would-fund-me, the-CIA-is-suppressing-it, utterly bizarre theory about quantum mechanics:
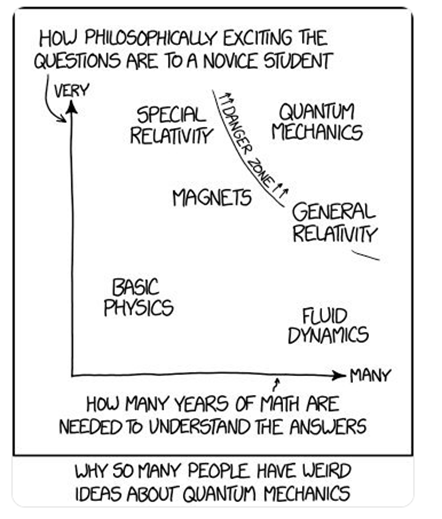
Only this week I deleted an email along those lines that I just couldn’t face responding to. Quantum Mechanics does, indeed, take a lot of maths to get to grips with in detail. In fact, it’s developed it’s own maths, in a sense. Try combining two particles of spin 1/2 and you get the result 0 and 1. Half plus half = zero and one. Logical? Well, if you do the tensor algebra, then yes. But for someone new to the subject, it is one of those very odd things about quantum mechanics that seems to make no sense.
Quantum mechanics is also tied-up with measurement. The act of measuring something, which is rather important in physics, has a special philosophical place in quantum mechanics (Just search for Quantum Measurement and Philosophy on YouTube and take your pick of results.) You can’t measure something without influencing it. (Although there is scope to be clever here – see the quantum bomb problem)
Now, one might also ask a converse question. Can you influence something without measuring it? This question I think is just as interesting. For example, our previous prime minister came in for some flak on his efforts to reduce child poverty when it emerged that New Zealand didn’t have any effective way of measuring child poverty. (So how do you know that your interventions have or haven’t worked, then? Does ‘reducing child poverty’ even mean anything if you can’t say what child poverty is?) And from a more mundane, in-my-lab-perspective, I’ve been grappling with a rather-less-serious-but-just-as-thorny problem of measuring small electric fields.
One can buy electric field meters, but these are hand-held things that are suitable for measuring the electric field strength in a thunderstorm or around a power lines, where fields can be quite large. For example, in a thunderstorm, the static electric field might build up to a few thousand volts per metre. But in my case I’m looking at fields produced by medical devices for stimulating the human body, for example transcranial magnetic stimulation, but with much smaller coils than normally used. These devices create small, localized electric fields, that are thought to stimulate neurons and neural pathways. How big are these fields? Well, for my coils maybe a few tens of volts per metre, spread over a length scale of a few millimetres. And they are really hard to measure.
So does that mean there’s no point to my work? Well, no, because even if we can’t reliably measure the strengths of the fields, we can measure the effects that these fields have. It’s a bit like saying that while child poverty itself might be hard to measure, the effect it has is not so hard to measure. In that sense, the ability (or lack of ability) to measure something may not be as limiting as it might appear.
So what has this to do with risk, you might ask? – Well it’s rather like the risk matrix with axes likelihood and impact. A bit like this: